Potential Energy, Thermodynamics, and Kinetics

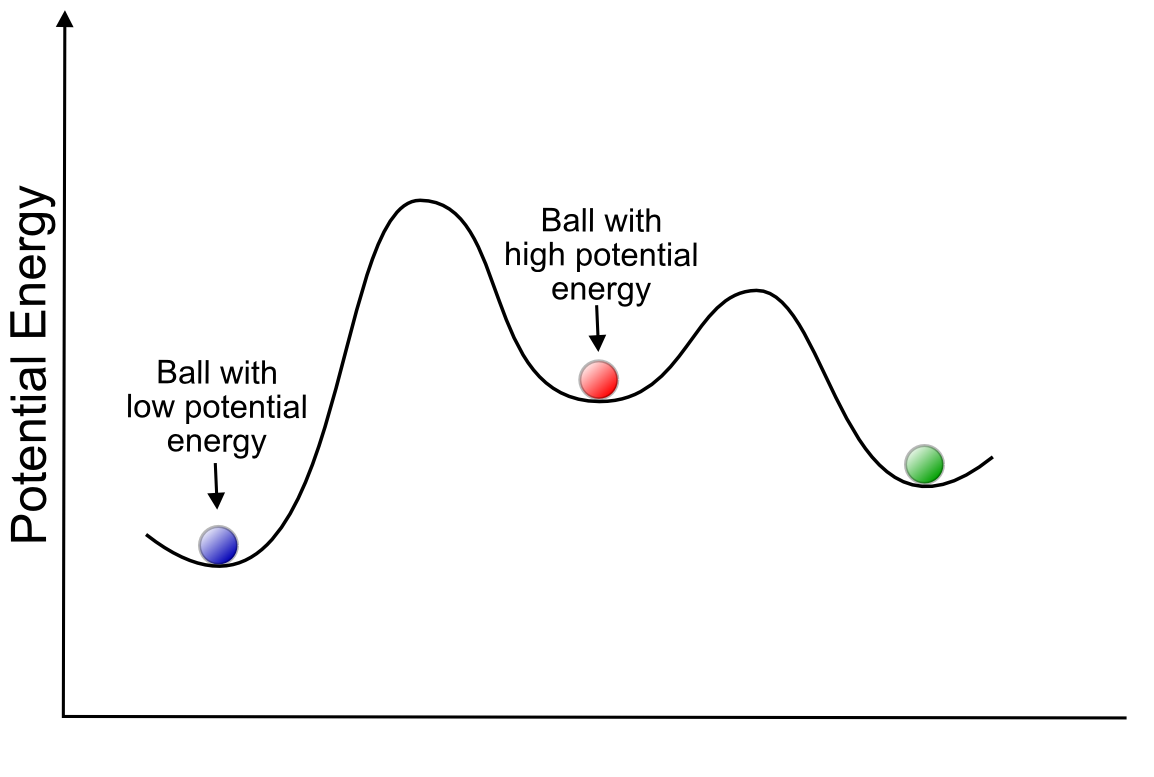
Potential energy is a concept that can be used to understand any type of change in a system. Any system (a collection of molecules, atoms, or electrons) has a potential energy associated with it. The origin of this energy and what it represents is not important, all that you need to know we can use the potential energy of a molecule to understand how and why it will undergo a change. These changes could include the formation or breaking of a bond, the gain or loss of an electron, or the change in physical orientation of the atoms in the molecule. Each physical change has an associated change in potential energy. If the molecule has a higher potential energy than it began with after the physical change, then it required energy input in order to complete the transformation, and we call this an endothermic transformation. If the molecule has a lower potential energy as a result of the transformation, then it released energy in the process, and we call this an exothermic transformation. In general, systems tend to change in ways that minimize potential energy. One of the more common analogies that is useful in helping to visualize this tendency is the example of a ball in a series of hills and valleys of different heights, where its potential energy is increased as its altitude increases, and decreased with a decrease in altitude.

hover over pic for description

Thinking in terms of the potential energy of molecular transformations, the valleys in this landscape represent stable molecules, and the peaks represent transition states between them. Our ball would like to find the lowest possible valley, but if there is a tall peak in its way, it will require a lot of energy to get there. For example, the "lowest valley" for a carbon atom in a piece of paper is as carbon dioxide (the product of burning it), but in order to do that, it would have to disconnect from all the carbon and hydrogen atoms around it. Not only does carbon have to "disconnect" from all its surrounding atoms, but the double bond between the two oxygen atoms of oxygen gas (O₂) has to break as well. These atoms are relatively stable connected to their current neighbors and a lot of energy needs to be put into the system in order to break these bonds. We should feel lucky that there is such a high energy barrier for this transformation, or else all organic material (including us!) would spontaneously combust.

In our hill-and-valley metaphor, the act of "putting energy into the system" is represented as expending energy to push the ball out of one stable valley and up a hill. After reaching the top of the hill, the ball begins to roll down the hill and release potential energy. In the case of combustion, CO₂ is a much more stable molecule and its "valley" would be much lower than that of the carbon in wood. So when the C and O atoms recombine and the metaphorical ball rolls down the hill toward its favored state (CO₂), so much energy is released that neighboring carbon and oxygen atoms are knocked out of their "valleys" up over the hill and on their way to CO₂, setting off a chain reaction.

hover over pic for description

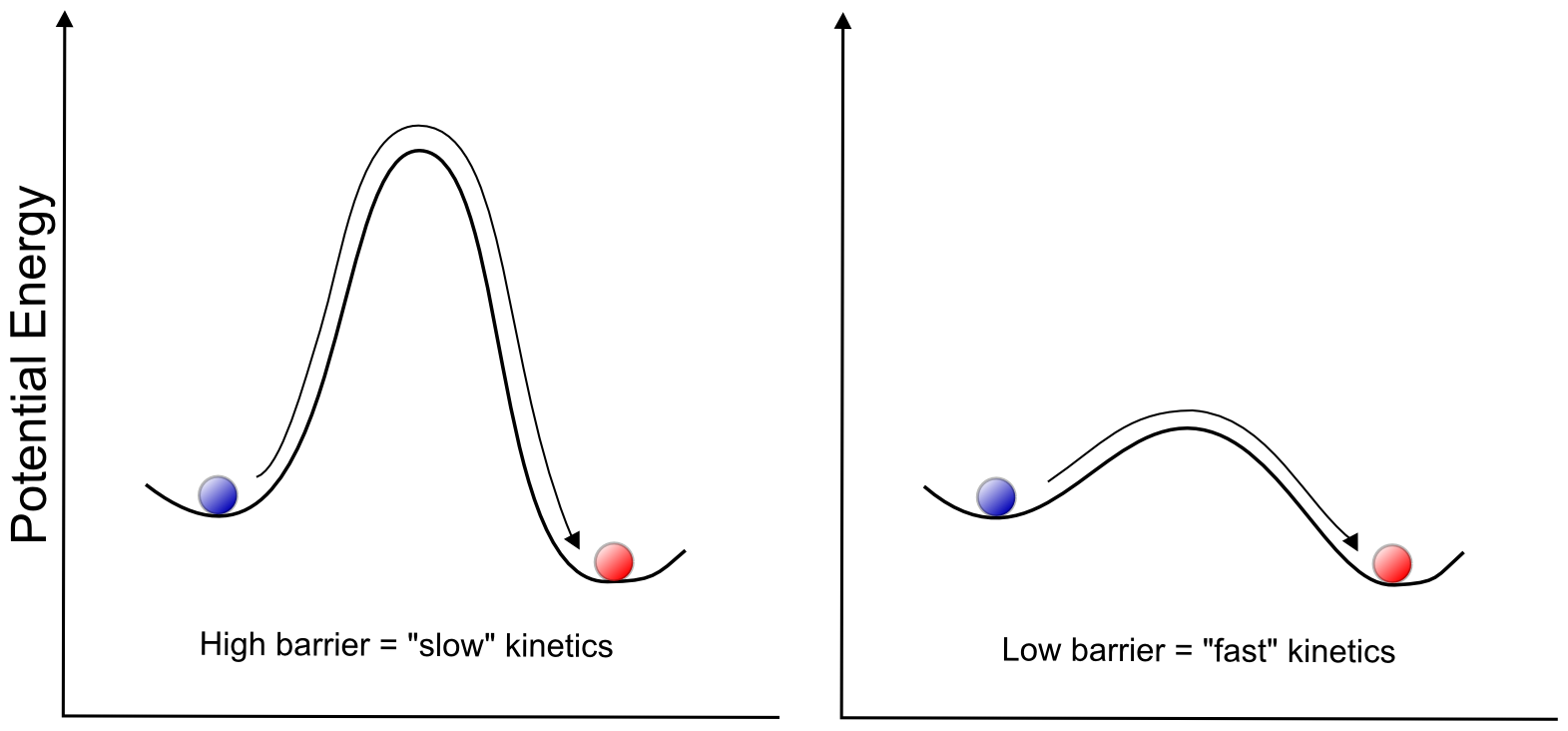
The thermodynamics of a change in a system is concerned with the difference in potential energy between two valleys, which allows us to determine whether a chemical transformation is energetically favorable (exothermic) or unfavorable (endothermic). The kinetics of a change in a system is trying to understand the barriers (hill peaks) between the valleys, and at what rate the system can transfer from one valley to another. In terms of electron transfer at an electrode, the thermodynamics of the transformation (gaining or losing an electron, and associated chemical changes) determines the E_1/2 of a wave, whereas the kinetics of the transformation determines the electrochemical reversibility of the wave. For example, a transformation with a high barrier (hill peak) between two valleys would result in a "slow" electron transfer and an irreversible wave, but a low barrier results in "fast" electron transfer and a reversible wave.

hover over pic for description

• Previous Section - Testing for Adsorption
• Next Section - Electrochemical Potential